Решите неравенство: (x^2-2x)^2-2*(x^2-2x)-3<=0.

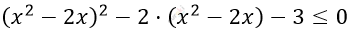
\[t = x^{2} - 2x\]

\[t^{2} - 2t - 3 \leq 0\]

\[(t - 3)(t + 1) \leq 0\]

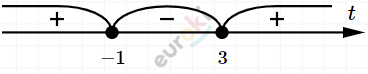
\[- 1 \leq t \leq 3 \Longrightarrow\]

\[\Longrightarrow - 1 \leq x^{2} - 2x \leq 3\]

\[\left\{ \begin{matrix} x^{2} - 2x \geq - 1 \\ x^{2} - 2x \leq 3\ \ \ \\ \end{matrix} \right.\ \text{\ \ \ \ \ \ \ \ }\]

\[\left\{ \begin{matrix} x^{2} - 2x + 1 \geq 0 \\ x^{2} - 2x - 3 \leq 0 \\ \end{matrix} \right.\ \text{\ \ \ \ \ \ \ }\]

\[\left\{ \begin{matrix} (x - 1)^{2} \geq 0\ \ \ \ \ \ \ \ \ \ \ \\ (x - 3)(x + 1) \leq 0 \\ \end{matrix} \right.\ \]

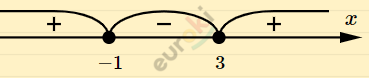
\[Ответ:\lbrack - 1;3\rbrack.\]