Решите систему уравнений: 6y^2-xy=2; x^2-5xy+3y^2=-1.

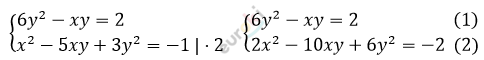
\[(1) + (2):\ 2x^{2} - 11xy + 12y^{2} =\]

\[= 0\]

\[\text{\ \ \ \ \ \ \ \ \ \ \ \ \ }(2x - 3y)(x - 4y) = 0\]

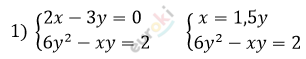
\[6y^{2} - 1,5y \cdot y = 2\]

\[6y^{2} - 1,5y^{2} = 2\]

\[4,5y^{2} = 2\]

\[y^{2} = \frac{4}{9}\]

\[y_{1} = \frac{2}{3};\ \ \ \ y_{2} = - \frac{2}{3}.\]

\[y_{1} = \frac{2}{3} \Longrightarrow \text{\ \ \ \ \ \ }x_{1} = 1,5 \cdot \frac{2}{3} = 1.\]

\[y_{2} = - \frac{2}{3} \Longrightarrow \text{\ \ \ \ \ }\]

\[\Longrightarrow x_{2} = 1,5 \cdot \left( - \frac{2}{3} \right) = - 1.\]

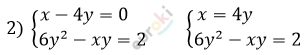
\[6y^{2} - 4y \cdot y = 2\]

\[6y^{2} - 4y^{2} = 2\]

\[2y^{2} = 2\]

\[y^{2} = 1\]

\[y_{3} = 1;\ \ \ \ y_{4} = - 1.\]

\[y_{3} = 1 \Longrightarrow \text{\ \ \ \ \ \ \ }x_{3} = 4 \cdot 1 = 4.\]

\[y_{4} = - 1 \Longrightarrow \text{\ \ \ \ }\]

\[\Longrightarrow x_{4} = 4 \cdot ( - 1) = - 4.\]

\[Ответ:\left( 1;\frac{2}{3} \right),\ \ \ \left( - 1;\ - \frac{2}{3} \right),\ \]

\[\text{\ \ }( - 4;\ - 1).\]