Вопрос:
Случайным образом выбирают одно из решений неравенства x^2-4x<=0. Какова вероятность того, что оно окажется и решением неравенства
Ответ:
|x-5|>=3?
\[x^{2} - 4x \leq 0\]
\[x(x - 4) \leq 0\]

\[|x - 5| \geq 3\]
\[\left\{ \begin{matrix} x - 5 \geq 0 \\ x - 5 \geq 3 \\ \end{matrix} \right.\ \text{\ \ \ \ \ \ \ \ \ \ \ \ }\text{\ \ \ \ }\left\{ \begin{matrix} x - 5 < 0\ \ \ \ \\ - x + 5 \geq 3 \\ \end{matrix} \right.\ \]
\[\left\{ \begin{matrix} x \geq 5 \\ x \geq 8 \\ \end{matrix} \right.\ \text{\ \ \ \ \ \ \ \ \ \ \ }\text{\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ }\text{\ \ }\left\{ \begin{matrix} x < 5 \\ x \leq 2 \\ \end{matrix} \right.\ \]
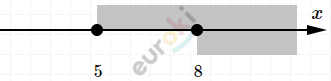
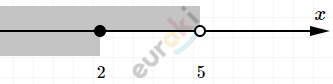
\[То\ есть\ длина\ отрезка\ = 4.\ \]
\[Совместим:\]

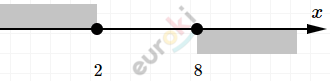
\[Общие\ решения:\ \ 3\ штуки.\]
\[Р = \frac{3}{4}.\]